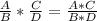Answer:
Option D.
Explanation:
we have
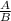 and
and
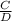
we know that
To multiply fractions multiply the numerators of the fractions to get the new numerator and multiply the denominators of the fractions to get the new denominator
To divide two fractions, multiply the first fraction by the reciprocal of the second fraction
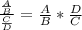
and