Question

The volumes of two similar figures are 27 mm3 and 1331 mm3. If the surface area of the smaller figure is 18 mm2, what is the surface area of the larger figure?

Answer 1

242 mm²

Explanation:

Given 2 similar figures with

ratio of sides = a : b, then

ratio of areas = a² : b² and

ratio of volumes = a³ : b³

Here the ratio of volumes = 27 : 1331, hence

ratio of sides =
`\sqrt[3]{27}` :
`\sqrt[3]{1331}` = 3 : 11, thus

ratio of areas = 3² : 11² = 9 : 121

let x be the surface area of the larger figure then by proportion

`(18)/(9)` =
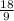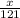`(x)/(121)` ( cross- multiply )

9x = 18 × 121 ( divide both sides by 9 )

x =
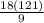`(18(121))/(9)` = 2 × 121 = 242

The surface area of the larger figure is 242 mm²