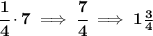Question

You need to determine the total distance each hiker will hike.

And


Determine the number off gallons of water each hiker will bring.

Explain

Use picture

Answer 1

so, on the way over the hikers will hike 2 miles, rest and then go the rest of 1¾ miles, meaning on the way over they'll hike 2 + 1¾ miles.

`\bf \stackrel{mixed}{1(3)/(4)}\implies \cfrac{1\cdot 4+3}{4}\implies \stackrel{improper}{\cfrac{7}{4}} \\\\[-0.35em] ~\dotfill\\\\ 2+\cfrac{7}{4}\implies \cfrac{2}{1}+\cfrac{7}{4}\implies \cfrac{(4)2+(1)7}{4}\implies \cfrac{8+7}{4}\implies \cfrac{15}{4}`

then on the way back, we know is -1/2 less than on the way over, that means the way back is (15/4) - (1/2)

`\bf \cfrac{15}{4}-\cfrac{1}{2}\implies \cfrac{(1)15-(2)1}{4}\implies \cfrac{13}{4} \\\\[-0.35em] ~\dotfill\\\\ \stackrel{\textit{total hiked distance}}{\stackrel{\textit{on the way over}}{\cfrac{15}{4}}+\stackrel{\textit{on the way back}}{\cfrac{13}{4}}}\implies \cfrac{(1)15+(1)13}{4}\implies \cfrac{28}{4}\implies 7`

we know is 1/4 for 1 mile, than how many for 7 miles?, well is just their product

`\bf \cfrac{1}{4}\cdot 7\implies \cfrac{7}{4}\implies 1(3)/(4)`