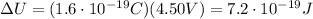Answer:
Step-by-step explanation:
The change in electrical potential energy of a charged particle moving through a potential difference is given by

where
q is the magnitude of the charge of the particle
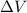 is the potential difference
is the potential difference
In this problem:
- the charge of the particle is 3.00 elementary charges, so
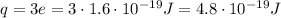
- the potential difference is
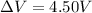
So, the change in electrical potential energy is