Question

Prove tha 15+17root3 is an irrational number

Answer 1

First of all, let's make the following observations:

15 is an integer, so in particular it is rational. The sum of two rational is rational. This means that
`15+17√(3)` is irrational if and only if
`17√(3)` is irrational.

Similarly, since 17 is rational and the multiplication of two rationals is rational, we have that
`17√(3)` is irrational if and only if
`√(3)` is irrational.

The proof that
`√(3)` is irrational is the following: suppose by contradiction that we could write

`√(3)=(a)/(b)`

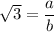
where a and b are two integers with no common factors.

Squaring both sides, we have

`(a^2)/(b^2)=3 \iff a^2 = 3b^2`

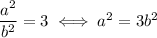
So,
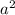`a^2` is a multiple of 3. This happens if and only if a itself is a multiple of 3. So, we can write
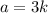`a=3k` and the expression becomes

`(3k)^2 = 3b^2 \iff 9k^2 = 3b^2 \iff 3k^2 = b^2`

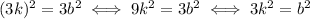
So,
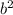`b^2` is a multiple of 3 as well, and this happens if and only if b is itself a multiple of 3.

So, we started with the assumption that we could write
`√(3)` as a reduced fraction, but this assumption led to a contradiction.

We deduce that
`√(3)` is irrational, and so are
`17√(3)` and
`15+17√(3)`