Question

Help with this needed

Answer 1

`12x ^(2)-7x -= 0 \\ \\ x = (7 + √(481) )/(24) \: or \: x = (7 - √(481) )/(24) \\ \: b. \: -7 \\ \\3x^(2) + 3x + 2 = 0 \\ c. \: no \: real \: solutions`

Answer 2

1. -7

2. no real solution

Step-by-step explanation

1. The given quadratic equation is:

`12 {x}^(2) - 7x - 9 = 0`

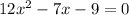
Comparing this to

`a{x}^(2) + bx + c= 0`

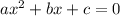
we have a=12, b=-7 and x=-9.

Therefore the value of b is -7

2. The given quadratic equation is

`3{x}^(2) + 3x + 2= 0`

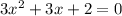
We have a=3,b=3 and c=2.

The discriminant of this equation is

`D= {b}^(2) - 4ac`

`D= {3}^(2) - 4(3)(2)`

`D= 9- 24 = - 15`

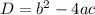
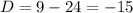
Since the discriminant is negative, the equation has no real roots.