Hello!
The answer is:
The simplified expression is:
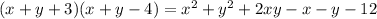
Why?
To solve the problem, we need to remember how to use the distributive property and how to add like terms.
The distributive property can be defined as follow:
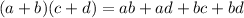
The like terms are the terms that share the same variable and the same exponent, for example:
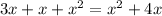
We were able to add the first and the second term because they share the same variable and the same exponent.
Now, we are given the expression:
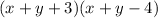
So, simplifying we have:
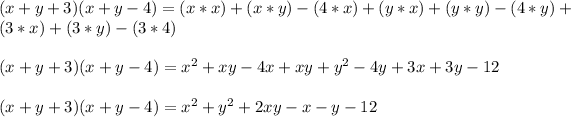
Hence, we have that the simplified expression is:
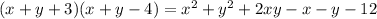
Have a nice day!