ANSWER
1. No real roots
2.

3. The discriminant is negative.
Step-by-step explanation
1. The given equation is
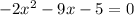
We have a=-2,b=-9 and c=-5.
The discriminant is given by:
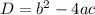
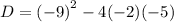
This simplifies to:

Since the discriminant is less than zero, the quadratic equation has no real roots.
2. The given equation is:
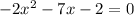
We have a =-2, b=-7 and c=-2.
The roots of this equation are given by;
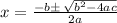
We plug in the values to get;
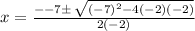
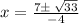
3. The given graph is hanging downwards. This means that it doesn't have x-intercepts.
Therefore the roots are complex or imaginary.
This implies that, the discriminant of the corresponding equation is negative.