Answer:
3544
Explanation:
This is a problem of compound growth. The formula is
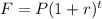
Where F is the value in the future (in this case, the population after 13 years)
P is the intial amount (here, the initial population of 2000, so P = 2000)
r is the rate of growth (here, it is 4.5%, in decimal, 0.045)
t is the time frame (here, it is 13 years, so t = 13)
we can plug the numbers into the formula and solve for F:
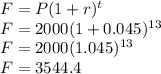
rounded to the nearest whole number, the population after 13 years would be 3544