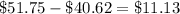Question

Jim would like to create a pencil holder with no top. He would like it to be 5 inches taller and 3 inches wide. He can't decide if he would like to make it a square base or a circular base. If the material costs $0.75 per square inch, how much more would it cost him to make a square prism than a cylinder?

Answer 1

`\$11.13`

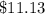
Explanation:

step 1

Find the surface area of the cylinder

The surface area of the cylinder is equal to

`SA=\pi r^(2) +2\pi rh`

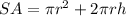
we have

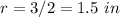`r=3/2=1.5\ in` ----> the radius is half the diameter

`h=5\ in`

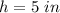
assume

`\pi =3.14`

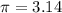
substitute

`SA=(3.14)(1.5)^(2) +2(3.14)(1.5)(5)=54.165\ in^(2)`

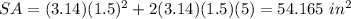
Find the cost

`54.165*(0.75)=\$40.62`

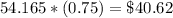
step 2

Find the surface area of the square prism

The surface area of the prism is equal to

`SA=b^(2) +4bh`

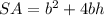
we have

`b=3\ in`

`h=5\ in`

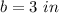
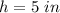
substitute

`SA=(3)^(2) +4(3)(5)=69\ in^(2)`

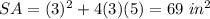
Find the cost

`69*(0.75)=\$51.75`

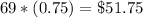
step 3

Find the difference of costs

`\$51.75-\$40.62=\$11.13`