Check the picture below.
so the domain will be the values that "x" gets, now, the maximum height of the ball is when it reaches the vertex or U-turn up above, well, what is the x-coordinate anyway?
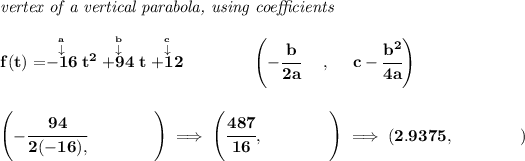
so then x = 2.9375 at the vertex, now, what is "x" when it hits the ground? recall y = 0 at that instant.
![\bf \stackrel{f(t)}{0}=-16t^2+94t+12\implies 0=-2(8t^2-47t-6) \\\\\\ 0=(8t+1)(t-6)\implies t= \begin{cases} \boxed{6}\\ \begin{matrix} -(1)/(8) \\[-0.5em]\cline{1-1}\\[-5pt]\end{matrix} \end{cases}](https://img.qammunity.org/2020/formulas/mathematics/middle-school/9720qqqy1iot6srw8gut1q2okym182wswq.png)
so then, the values for "x" or namely the domain from the vertex till the ball hits the ground is 2.9375 < t < 6.