Question

A bag contains different colored candies. There are 50 candies in the bag, 28 are red, 10 are blue, 8 are green and 4 are yellow.

What is the probability of choosing five pieces of candy and getting 2 red and 3 green?

Answer 1

`\displaystyle (54)/(5405)`.

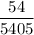Explanation:

How many unique combinations are possible in total?

This question takes 5 objects randomly out of a bag of 50 objects. The order in which these objects come out doesn't matter. Therefore, the number of unique choices possible will the sames as the combination

`\displaystyle \left(50\atop 5\right) = 2,118,760`.

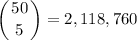How many out of that 2,118,760 combinations will satisfy the request?

Number of ways to choose 2 red candies out a batch of 28:

`\displaystyle \left( 28\atop 2\right) = 378`.

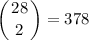Number of ways to choose 3 green candies out of a batch of 8:

`\displaystyle \left(8\atop 3\right)=56`.

However, choosing two red candies out of a batch of 28 red candies does not influence the number of ways of choosing three green candies out of a batch of 8 green candies. The number of ways of choosing 2 red candies and 3 green candies will be the product of the two numbers of ways of choosing

`\displaystyle \left( 28\atop 2\right) \cdot \left(8\atop 3\right) = 378* 56 = 21,168`.

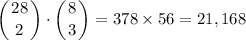The probability that the 5 candies chosen out of the 50 contain 2 red and 3 green will be:

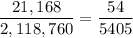`\displaystyle (21,168)/(2,118,760) = (54)/(5405)`.