Answer:
89.0°
Explanation:
The segment from the centre of the circle to the chord is a perpendicular bisector, hence
third side of right triangle = 12.7 ÷ 2 = 6.35
The angle subtended at the centre by arc CD is twice the angle subtended by the right triangle at the centre
The triangle from the centre to C is congruent to the triangle from the centre to D
Calculate the angle (Θ ) in the right triangle using the sine ratio
sinΘ =
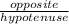 =
=

Θ =
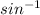 (
(
 ) ≈ 44.5°
) ≈ 44.5°
Hence
measure of CD = 2 × Θ = 2 × 44.5 = 89.0°