Answer:
(a) There will be no water in the bucket by the time the bucket reaches the top of the well.
(b) The work done in this process will be approximately 1.98 × 10³ J.
Step-by-step explanation:
(a)
How long will it take for the bucket to reach the top of the well?
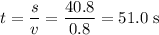 .
.
How much water will leak out during that
 seconds?
seconds?
 .
.
That's more than all the water in the bucket. In other words, all
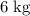 of water in the bucket will have leaked out by the time the bucket reaches the top of the well. There will be no water in the bucket by the time the bucket reaches the top of the well.
of water in the bucket will have leaked out by the time the bucket reaches the top of the well. There will be no water in the bucket by the time the bucket reaches the top of the well.
(b)
How long will it take for all water to leak out of the bucket?
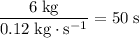 .
.
In other words,
- For the first 50 seconds, water will leak out of the bucket. The mass of the bucket will decrease from
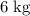 to
to
 at a constant rate.
at a constant rate. - For the last 1 second, the mass of the bucket will stay constant at
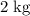 .
.
Express the mass
 of the bucket about time
of the bucket about time
 as a piecewise function:
as a piecewise function:
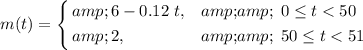 .
.
Gravity on the bucket:
 .
.
However, the bucket is moving at a constant velocity. There's no acceleration. By Newton's Second Law, the net force will be zero. Forces on the bucket are balanced. As a result, the size of the upward force shall be equal to that of gravity.
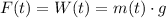 .
.
The speed of the bucket
 is constant. Thus the power
is constant. Thus the power
 that pulls the bucket upward at time
that pulls the bucket upward at time
 will be:
will be:
 .
.
Express work as a definite integral of power with respect to time:
 .
.
Both
 and
and
 here are constants. Factor them out:
here are constants. Factor them out:
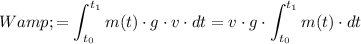 .
.
Integrate the piecewise function
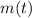 piece-by-piece:
piece-by-piece:
![\displaystyle \begin{aligned}W &= v\cdot g\cdot \int_(t_0)^(t_1){m(t)\cdot dt}\\ &=0.8* 9.8 \left [\int_(0)^(50)(8-0.12\;t)\cdot dt + \int_(50)^(51)2\cdot dt\right]\\ &=0.8* 9.8 \left [\left(8\;t - (0.12)/(2)\;t^(2)\right)\bigg|^(50)_(0) + (2\;t)\bigg|^(51)_(50)\right] \\&=0.8* 9.8 \left [\left(8* 50-(0.12)/(2)* 50^(2)\right)+ (51* 2 - 50* 2)\right]\\&=\rm 1975.68\;J \end{aligned}](https://img.qammunity.org/2020/formulas/physics/college/etj3sdnvdwcb2p6bk78u9fz7qzou9og0dz.png) .
.
Hence the work done pulling the bucket to the top of the well is approximately
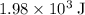 .
.