Question

A sphere has a surface area of 36π ft2. Find the volume of the sphere.

36π ft3


42π ft3


48π ft3


28π ft3

Answer 1

The surface area of the sphere is given by the equation

`A=4\pi * r^(2)`,

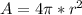where A is the surface area and r is the radius.

We want to find the volume of the sphere, which is given by the equation

`V = (4)/(3) * \pi * r^(3)`,

where V is the volume and r is the radius.

Looking at these equations, we see that they both involve the sphere's radius. If we know what r is, we can calculate the volume.

We know that the sphere's surface area is
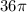`36 \pi`. Plugging that in for A in the surface area equation, we get

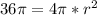`36 \pi=4\pi * r^(2)`, then divide by
`\pi`

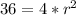`36 = 4 * r^(2)`, then divide by 4

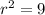`r^(2) = 9`, then take the square root of both sides

`r = 3`

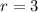
So the radius of the sphere is 3. Plugging this into the volume equation,

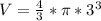`V = (4)/(3) * \pi * 3^(3)`, simplify terms

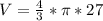`V = (4)/(3) * \pi * 27`, multiply
`(4)/(3)` by 27

`V = 36 * \pi`

So the volume of the sphere is
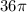`36\pi`.

Answer 2

36π ft^3

Explanation:

The surface area (S) of a sphere can be defined as:

S = 4×π×r^2 = 36×π

Solve for r to get the radius of the sphere:

r = (
`√(36/4)` = 3

The voluem (V) of a sphere can be defined as:

V= (4/3)×π×r^3

The volume of the sphere is:

V = (4/3)×π×(3^3) = 36π ft^3

The volume of the sphere can be calculated from the surface area given.