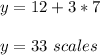Question

(HELP ASAP PLEASE)

Band students are tested on, and required to pass, a certain number of scales during the year. As of today, Nick has passed 5 scales, whereas his friend Mary has passed 12 of them. Going forward, Nick has committed to passing 4 scales per week, and Mary has committed to passing 3 per week. At some point soon, the two friends will have passed the same number of scales. How many scales will that be? How long will that take?


The friends will each have passed ___ scales in ___ weeks.

Answer 1

The friends will each have passed _33_ scales in _7_ weeks.

Explanation:

We must write an equation for the number of scales that Nick approves and an equation for the number of scales that Mary approves.

For Nick we have:

5 initial scales

Nick has committed to approve 4 scales per week. Let's call x the number of weeks.

So the number of scales and for Nick is:

`y = 5 + 4x`

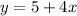
For Mary we have:

12 initial scales

Mary has committed to approve 3 scales per week. Let's call x the number of weeks.

So the number of scales and for Mary is:

`y = 12 + 3x`

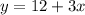
To know when the two friends have passed the same number of scales we must equal both equations and solve for x:

`12 + 3x = 5 + 4x`

`12-5= 4x-3x`

`x= 7\ weeks`

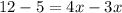
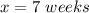
And

`y=12+3*7\\\\y=33\ scales`