Question

Can someone please help me with this problem

Answer 1

294.5 sq meters

Explanation:

I found the area of the circle, subtracted away the area of the sector, then had to add back in the area of the triangle. The areas for each is as follows:

`A_(c)=\pi (11.1)^2`

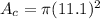
A = 387.0756 sq m

`A_(s)=(130)/(360)*\pi (11.1)^2`

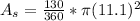
A = 139.7773

`A_(t)=(1)/(2)(11.1)(11.1)sin(130)`

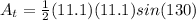
A = 47.1922

Now taking the area of the circle - area of sector + area of triangle:

387.0756 - 139.7773 + 47.1922 = 294.5 sq m