Question

Please help answer this geometry

Answer 1

a). (10x + 15y)² = (8x + 12y)² + (6x + 9y)²

b). It's an identity

Explanation:

a). Pythagoras theorem says

(Hypotenuse)² = Height² + Base²

Therefore, form the given picture

(10x + 15y)² = (8x + 12y)² + (6x + 9y)² will be the equation.

b). For an identity we have to prove Right hand side of the equation(R.H.S.) = Left hand side of the equation(L.H.S.)

Now we solve the L.H.S. of the equation

(10x + 15y)²

= 100x² + 225y² + 300xy [ (a + b)² = a²+ b² + 2ab

Now we solve R.H.S. of the equation

(8x + 12y)² + (6x + 9y)²

= 64x²+ 144y² + 36x² + 81y² + 108y + 192xy + 108xy

= 100x² + 225y²+ 300xy

Therefore, L.H.S. = R.H.S.

The given equation is an identity.

Answer 2

a.
`(8x+12y)^(2) +(6x+9y)^(2) = (10x+15y)^(2)`

b.
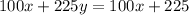`100x+225y=100x+225` Which means it is an identity

Explanation:

The Pythagorean Theorem states that in every right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the respective lengths of the legs. It is the best-known proposition among those that have their own name in mathematics.

If in a right triangle there are legs of length a, and b, and the measure of the hypotenuse is c, then The following relationship is fulfilled:

`a^(2) +b^(2)=c^(2)`

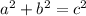
a. Write an equation using the Pythagorean Theorem and the measurements provided in the diagram

From the exercise shown in the image, we can get the values of a, b, and c.

a = 8x+12y, b = 6x+9y, and c = 10x+15y

Write an equation using the Pythagorean Theorem

We obtain:

`(8x+12y)^(2) +(6x+9y)^(2) = (10x+15y)^(2)`

b. Transform each side of the equation to determine if it is an identity.

In mathematics, an identity is the realization that two objects that are mathematically written differently, are in fact the same object. In particular, an identity is an equality between two expressions, which is true whatever the values ​​of the different ones are.

So, we transform each side of the equation

`(8x+12y)^(2) +(6x+9y)^(2) = (10x+15y)^(2)`

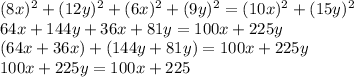`(8x)^(2) +(12y)^(2) +(6x)^(2) +(9y)^(2)= (10x)^(2)+(15y)^(2)\\64x+144y+36x+81y=100x+225y\\(64x+36x)+(144y+81y)=100x+225y\\100x+225y=100x+225` Which means it is an identity

.