Question

What is the height of the cone below?
- 3
- 9
- 27
- 75

Answer 1

`\bf \textit{volume of a cone}\\\\ V=\cfrac{\pi r^2 h}{3}~~ \begin{cases} r=radius\\ h=height\\ \end{cases}\implies V=\pi r^2\cdot \cfrac{h}{3}~~ \begin{cases} \pi r^2=&area~of\\ &circular\\ &base\\ \cline{1-2} \stackrel{B}{\pi r^2}=&5\\ V=&45 \end{cases} \\\\\\ 45=5\cdot \cfrac{h}{3}\implies 135=5h\implies \cfrac{135}{5}=h\implies 27=h`

Answer 2

The correct option is C) 27

Explanation:

Consider the provided figure.

Volume of a cone is:
`V=(1)/(3)\pi r^2h`

The area of base of the cone is = area of circle =
`\pi r^2`

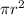
Volume of the cone is 45 in³ and the area of base is given as 5 in²

Therefore,
`45=(1)/(3)\pi r^2h`

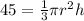
and
`5=\pi r^2`

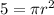
Substitute the value of
`\pi r^2=5` in
`45=(1)/(3)\pi r^2h`

`45=(1)/(3)* 5* h`

`45* (3)/(5)=h`

`h=27`

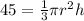
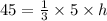
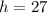
Hence, the height of the cone is 27 in.

Therefore, the correct option is C) 27