Question

Two consecutive perfect squares have a difference of $99$. what is the value of the larger perfect square?

Answer 1

2500

Explanation:

Let the first square number be
`x^2` then the next square number is
`(x+1)^2`.

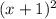The difference between these two consecutive numbers is 99.

This implies that:

`(x+1)^2-x^2=99`

We expand to get:

`x^2+2x+1-x^2=99`

Simplify:

`2x=99-1`

`2x=98`

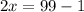
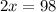
Divide both sides by 2

`x=49`

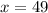
Therefore the value of the larger perfect number is

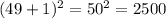`(49+1)^2=50^2=2500`.