Question

URGENT!!!!!!! PLZ HELP ME AS FAST AS U CAN!!!!!!!!!!!!

what are the soluttions of the equation x^2+4x+4=25?

a)x=3 or x=7

b)x=-3 or x=-7

c)x=1 or x=9

d)x=1 or x=-9

Answer 1

x^2 + 4x + 4 is the perfect square of (x + 2)

so x^2 + 4x + 4 = (x+2)^2

Substitute x^2 + 4x + 4 with (x+2)^2 in the equations:

(x + 2)^2 = 25

Notice that 25 is also the perfect square of 5 so 5^2 = 25

Substitue 25 with 5^2 in the equation:

(x + 2)^2 = 5^2

Since both sides of the equation are being squared, we can square root both sides, and since we re doing this to both sides, both sides are still equal and the equation still holds true

`\sqrt{( {x + 2) }^(2) } \: \: = \: \: \sqrt{ {5}^(2) }`

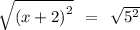
This results in:

+-(x + 2) = +- 5

This means the 5 can be either postivie or negative, so there are 2 sollutions:

x + 2 = 5 x + 2 = -5

x = 3 x = -7

Its 3 or -7 so you must ve copied the wrong possible answers