Question

Two cars started moving from San Jose to San Diego. The speed of the faster car was 12 mph less than twice the speed of the other one. In 6 hours the faster car got to San Diego, and by that time the slower one still was 168 miles away from the destination. Find their speeds.

Answer 1

460 miles to San Diego

460/6=76.6 repeating -12 then divided by 2

means the other slower car was going approx 32.3 MPH

I may be wrong considering i had to google how far san diego was, if there was a graph or anything telling distance lmk

Answer 2

Speed of the faster car is 68 mph and speed of slower car is 40 mph.

Explanation:

Let the faster car has the speed = x mph

and slower car has the speed = y mph

Let the distance between San Jose and San Diago = d miles

Now we will form the first equation.

"Speed of the faster car was 12 mph less than twice the speed of the slower one"

x = 2y -12 ----------(1)

Since "faster car cover the distance d in 6 hours"

so,
`x=(d)/(6)`

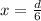
"By that time the slower car still was 168 miles away from the destination"

so,
`y=(d-168)/(6)`

`y=(d)/(6)-(168)/(6)`

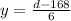
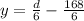
y = x - 28 {since
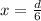`x=(d)/(6)`} ---------(2)

By substitution method,

x = 2(x - 28) - 12

x = 2x - 56 - 12

x - 2x = - 68

x = 68 mph

By putting x = 68 in equation 2

y = 68 - 28

y = 40 mph

Therefore, speed of the faster car is 68 mph and speed of slower car is 40 mph