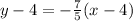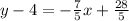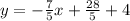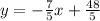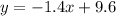Answer:
With fractions:
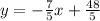
With decimals:
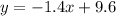
Explanation:
First we are finding the slope of line AB using the slope formula:

where
 is the slope
is the slope
 are the coordinates of the first point
are the coordinates of the first point
 are the coordinates of the second point
are the coordinates of the second point
The first point is A= (-3, -1) and the second one is B = (4, 4), so
 ,
,
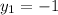 ,
,
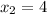 and
and
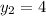 .
.
Replacing values
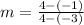
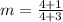
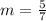
Now, since AB and BC form a right angle, BC is perpendicular to AB. The slope of a perpendicular line is the negative reciprocal of the other line; in other words, the slope of BC is the negative reciprocal of the slope of AB. To find the negative reciprocal of the slope of AB we just need to multiply its slope by -1 and invert the fraction:
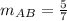
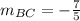
Finally, to complete the equation of BC, we are using the point-slope formula:

where
 is the slope
is the slope
 are the coordinates of the point
are the coordinates of the point
We know that
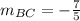 , so
, so
 . Since the line passes throughout point B = (4, 4),
. Since the line passes throughout point B = (4, 4),
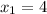 and
and
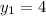 .
.
Replacing values