Question

Find the domain and range of the function.
f(x) = |x – 4| + 3

Answer 1

`f(x) = |x-4|+3 \\ \\ \text{We have no existential conditions: }\\ \Rightarrow D = \mathbb{R}\\ \\ |x-4|\geq 0~~\big|+3 \Rightarrow |x-4|+3 \geq 3 \Rightarrow f(x) \geq 3 \\ \\ \Rightarrow \text{The range of the function is }[3,+\infty)`

Answer 2

Domain:

All real numbers

Range:

`y \geqslant 3`

Step-by-step explanation

The given function is

`f(x) = |x - 4| + 3`

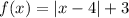
This is the function obtained by the shifting the base absolute value function to the right by 4 units and up by 3 units.

The vertex of this function is at:

(4,3)

The domain is all real numbers because there is no x-value that will make the function undefined.

The least y-value on this graph is 3.

The graph opens up forever.

The range is [3,∞) or y≥3