Question

in the system shown below, what are the coordinates of the solution that lies in quadrant IV? x2+y2=25 x-y2=-5

Answer 1

if you type (4,-3) is wrong

Answer 2

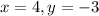`x=4,y=-3`, or as an ordered pair: (4, -3)

Explanation:

We have the system of equations

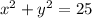`x^2+y^2=25` (1)

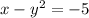`x-y^2=-5` (2)

Let's solve our system step-by-step:

Step 1. Solve for
`y^2` in equation (1)

`x^2+y^2=25`

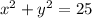
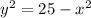`y^2=25-x^2` (3)

Step 2. Replace equation (3) in equation (2) and solve for
`x`

`x-y^2=-5`

`x-(25-x^2)=-5`

`x-25+x^2=-5`

`x^2+x-20=0`

`(x+5)(x-4)=0`

`x+5=0,x-4=0`

`x=-5,x=4`

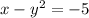
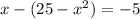
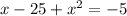
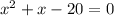
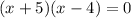
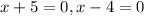
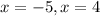
Remember that in the fourth quadrant x is positive and y is negative, so we are taking the positive value of x; in other words
`x=4` (4)

Step 3. Replace equation (4) (the positive value of x) in equation (1)

`x^2+y^2=25`

`4^2+y^2=25`

`16+y^2=25`

`y^2=25-16`

`y^2=9`

`y=√(9) ,y=-√(9)`

`y=3,y=-3`

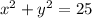
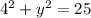
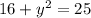
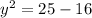
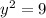
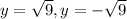
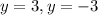
Since y is negative in quadrant IV, we take
`y=-3`

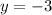
We can conclude that the solution of our system of equations that lies in the quadrant IV is
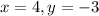`x=4,y=-3`, or as an ordered pair: (4, -3)