Question

If 11250 = m(600) + b and 7650 = m(400) + b, how do I find the values of b and m? I forgot how to compare when I have two different values. If someone can find me a lesson online or show work, that would be the best. I do not simply need answers, thank you.

Answer 1

`b=450` and
`m=18`

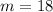
Explanation:

This exercise is an example of linear system equations. A system of linear equations is a set of (linear) equations that have more than one unknown. The unknowns appear in several of the equations, but not necessarily in all of them. What these equations do is relate the unknowns to each other.

The easy way to solve this problem is by using the reduction method.The reduction method consists of operating between the equations, such as adding or subtracting both equations, so that one of the unknowns disappears. Thus, we obtain an equation with a single unknown.

Ordering the equations as a system equations.

`\left \{ {{600m+b=11250} \atop {400m+b=7650}} \right.`

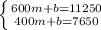
Using the reduction method, let's substract the second equation with the first equation, in order to clear m.

600m + b = 11250

-400m - b = -7650

200m = 3600 --------> m = 3600/200 -----> m = 18

We can substitute the value of m in any of the two equations to obtain the unknow b.

Let's substitute the value of m in the second equation.

400(18) + b = 7650

7200 + b = 7650 -----> b = 7650 - 7200 -------> b = 450

We can check this values in both equations.

600m + b = 11250 -----> 600(18) + 450 = 11250

400m + b = 7650 -----> 400(18) + 450 = 7650

Satisfying the result of both equations.