Question

What is the formula for the sum of the interior angles of a polygon

Answer 1

• The fomula for the sum of the interior angles of a polygon is:

Sum of the interior angles = (n - 2) × 180°.

Where n is the number of sides of the polygon.

Step-by-step explanation:

The formula (n - 2) × 180° is valid for any convex polygon.

A convex polygon is one whose interior angles (every interior angle) measure less than 180°.

You can prove and remember that formula following this reasoning:

• If you pick one vertex of the polygon you can build (n - 2) diagonals, and so split the figure into n - 2 triangles.

• Since, the sum of the interior angles of any trianle is 180°, the sum of the total angles is (n - 2) × 180°. And this is the formula for the sum of the interior angles of a polygon.

For example, for a pentagon, a polygon with 5 sides, you can can draw 5 - 2 = 3 diagonals from one vertex, and so obtain 3 triangles. Then the sum of the interior angles shall be (n - 2) × 180° = (5 - 2) × 180° = 3 × 180° = 540°.

Answer 2

Sum of the interior angles = (n-2) x 180°

where

n is the number of sides of the polygon

Step-by-step explanation:

The formula for the sum of the interior angles of a polygon is:

`sum=(n-2)*180`

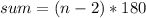
where

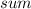`sum` is the sum of the interior angle of the polygon

`n` is the number of polygons

Let's check the formula using an example:

We want to find the sum of the interior angles of a square, we know that a square has 4 sides, so
`n=4`.

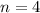Replacing values

`sum=(4-2)*180`

`sum=(2)*180`

`sum=360`

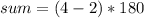
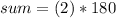
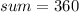
We can apply the same procedure to any convex polygon with n sides.