Question

What is the equation for the translation of x2 + y2 = 16 seven units to the right and five units up?

(x + 7)2 + (y – 5)2 = 16
(x - 7)2 + (y + 5)2 = 16
(x + 7)2 + (y + 5)2 - 16
(x - 72 + (y – 5)2 = 16

Answer 1

`(x-7)^2+(y-5)^2=16`.

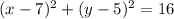Explanation:

The given circle has equation
`x^2+y^2=16`.

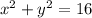This is the equation that has its center at the origin with radius 4 units.

When this circle is translated seven units to the right and five units up, then the center of the circle will now be at (7,5).

The equation of a circle with center (h,k) and radius r units is
`(x-h)^2+(y-k)^2=r^2`.

This implies that, the translated circle will now have equation.

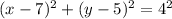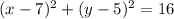`(x-7)^2+(y-5)^2=4^2`.

`(x-7)^2+(y-5)^2=16`.