Question

It will take Adam four hours to drive to Disney Park, and 2.5 times less time if driving 45 mph faster. What is the distance Adam should cover to get to the park? Pease and fanks

Answer 1

Hello!

The answer is:

The distance that Adam should cover to get to the park is 108 miles.

Why?

To solve the problem, we need to write two equations with the given information about the times and his speed.

So,

For the first equation we have: Going to Disney Park

`time=4hours`

`Distance=v*4hours`

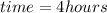
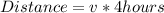
For the second equation we have: Going back from Disnery Park

`time=4hours-2.5hours=1.5hours`

`Speed=v+45mph`

`Distance=(v+45mph)*1.5hours`

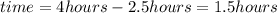
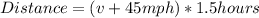
Now, if He covered the same distance going and coming back, we have:

`v*4hours=(v+45mph)*1.5hours`

`v*4hours=v*1.5hours+45mph*1.5hours`

`v*4hours-v*1.5hours=45mph*1.5hours`

`v*2.5hours=67.5miles`

`v=(67.5miles)/(2.5hours)=27(miles)/(hour)=27mph`

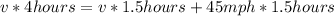
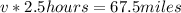
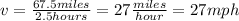
We have that the speed when Adam was going to Disney Park was 27 mph.

Therefore, to calculate the distance, we need to substitute the obtained speed in any of the two first equations.

Then, substituting the speed into the first equation, we have:

`Distance=v*4hours\\Distance=27mph*4hours=108miles`

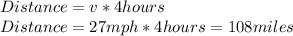
Hence, we have that the distance that Adam should cover to get to the park is 108 miles.

Havea nice day!