Answer:
At the beginning
16 men and 24 women
In the end
16 men and 20 women
Explanation:
Call x the number of men on the bus and call y the number of women on the bus.
We know that the initial ratio between men and women is 2: 3
And the final ratio is 4: 5
So
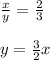 (1)
(1)
After the 4 women are down, the proportion is:
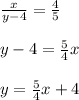 (2)
(2)
Substitute the value of y in the first equation and solve for x
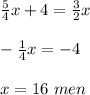
Now solve for y.
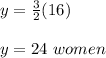
Then at the end there were 20 women (because 4 women got off the bus)