Question

Determine if the Mean Value Theorem for Integrals applies to the function f of x equals 2 times the square root of x on the interval [0, 4]. If so, find the x-coordinates of the point(s) guaranteed to exist by the theorem.

No, the theorem does not apply.
Yes, x = 1.
Yes, x equals 8 over 3.
Yes, x equals sixteen divided by 9.

Answer 1

`f(x)=2\sqrt x` is continuous on [0, 4] and differentiable on (0, 4), so the MVT holds. We have

`f'(x)=\frac1{\sqrt x}`

so that by the MVT, there is some
`c\in(0,4)` such that

`f'(c)=(f(4)-f(0))/(4-0)\implies\frac1{\sqrt c}=\frac{2\sqrt4}4=1`

`\implies1=\sqrt c\implies \boxed{c=1}`

Answer 2

x= 16/9

Explanation:

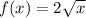`f(x) = 2√(x)` is differentiable on [0,4] so the Mean Value Theorem For Integrals applies.

Average Value of the Integral:

`(1)/(b-a) \int\limits^b_a {f(x)} \, dx = (1)/(4-0) \int\limits^4_0 {2√(x) } \, dx`

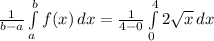
After evaluating you will get
`(8)/(3)`

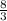
Now, this is the average value so you still need to find the x-value using the original equation:

`(8)/(3) =2√(x)\\\\(4)/(3) = √(x)\\\\(16)/(9) =x`