Question

Brayden is simplifying the expression 4n – (n – 3)2. He begins by distributing the negative to the terms inside the parentheses. What should he have done instead?

Answer 1

He should have simplified
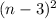`(n-3)^2` first then distribute the negative sign.

Explanation:

Given that Brayden is simplifying the expression
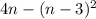`4n-(n-3)^2`. He begins by distributing the negative to the terms inside the parentheses. Which is basically the wrong step for the given problem.

Now we need to find about what should he have done instead.

According to order of operations, we should begin with exponent first.

So that means he should have simplified
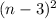`(n-3)^2` first then distribute the negative sign.

Answer 2

Brayden should have expanded the parenthesis first.

EXPLANATION

The expression Brayden is trying to simplify is

`4n - {( n - 2)}^(2)`

To simplify this expression, the first thing to do is to expand the perfect square to obtain:

`4n - {( n}^(2) - 4n + 4)`

We can now distribute the negative to the terms in the parenthesis.

`4n - { n}^(2) + 4n - 4`