Question

A single-slit diffraction pattern is formed on a distant screen. If the width of the single slit through which light passes is reduced, what happens to the width of the central bright fringe? Assume the angles involved remain small.

Answer 1

The width of the central bright fringe will increase

Step-by-step explanation:

In a single-slit diffraction pattern, the distance of the nth-minimum from the central maximum on the screen is given by

`y=(n \lambda D)/(d)`

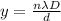
where

`\lambda` is the wavelength of the wave

D is the distance of the screen from the slit

d is the width of the slit

The width of the central bright fringe is equal to twice the value of y=1 (first minimum), so:

`w=2 y(n=1) = (2\lambda D)/(d)`

And we see that it is inversely proportional to the width of the slit: therefore, if the width of the slit is reduced, the width of the central brigh fringe will increase.