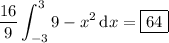In the
 -
-
 plane, the base has equation(s)
plane, the base has equation(s)
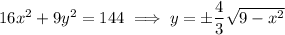
which is to say, the distance (parallel to the
 -axis) between the top and the bottom of the ellipse is
-axis) between the top and the bottom of the ellipse is
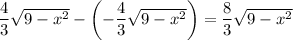
so that at any given
 , the cross-section has a hypotenuse whose length is
, the cross-section has a hypotenuse whose length is
 .
.
The cross-section is an isosceles right triangle, which means the legs occur with the hypotenuse in a ratio of 1 to
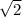 , so that the legs have length
, so that the legs have length
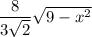 . Then the area of each cross-section is
. Then the area of each cross-section is
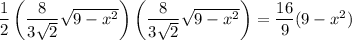
Then the volume of this solid is