Question

Object 1 and Object 2 have both the same mass. If object 1 moves twice as fast as Object 2, compare their kinetic energy.

A.) Object 1 has 1/4 the kinetic energy of object 2

B.) Object 1 has 1/2 the kinetic energy of object 2

C.) Object 1 has 2x the Kinetic energy of Object 2

D.) Object 1 has 4x the kinetic energy of object 2

Answer 1

D.) Object 1 has 4x the kinetic energy of object 2

Step-by-step explanation:

Kinetic Energy

Is the type of energy an object has due to its state of motion. It is proportional to the square of the speed.

The formula for the kinetic energy is:

`\displaystyle K=(1)/(2)mv^2`

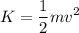
Where:

m = mass of the object

v = speed at which the object moves

Now suppose we have two objects with the same mass m1=m2=m and object 1 moves twice as fast as object 2, that is:

`v_1=2v_2`

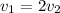
Let's compute their kinetic energies:

`\displaystyle K_1=(1)/(2)mv_1^2`

`\displaystyle K_2=(1)/(2)mv_2^2`

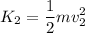
Since v1=2v2, the first kinetic energy is:

`\displaystyle K_1=(1)/(2)m(2v_2)^2`

`\displaystyle K_1=4(1)/(2)m(v_2)^2`

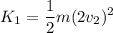
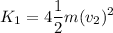
Dividing both equations:

`\displaystyle (K_1)/(K_2)=(4(1)/(2)m(v_2)^2 )/((1)/(2)m(v_2)^2)`

Simplifying:

`\displaystyle (K_1)/(K_2)=4`

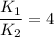
Or, equivalently:

`K_1=4K_2`

Answer:

D.) Object 1 has 4x the kinetic energy of object 2