Answer:

Explanation:
The numbers must be in the ratio 2:4:5:6.
Let's call them 2x, 4x, 5x, and 6x. Then
5x + 6x = 11x = sum of last two numbers
2x + 4x = 6x = sum of first two numbers
According to the condition,
11x – 6x = 4.8
5x = 4.8
x = 0.96
2x = 1.92; 4x = 3.84; 5x = 4.80; 6x = 5.76
The numbers are
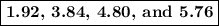
Check:
(4.80 + 5.76) – (1.92 + 3.84) = 4.8
10.56 – 5.76 = 4.8
4.8 = 4.8
OK.