Answer:
x=3, y=5 This means the point would be (3,5)
Explanation:
As I am unable to see your work, I am unable to tell you what you did wrong, but I hope you can notice what was wrong by comparing your work to mine
We are given the equations
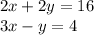
The first thing we need to do is solve one of them for a variable and use substitution. I chose the second equation
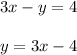
Now we can substitute in this equation for y
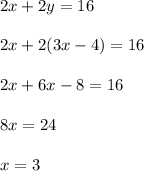
Now that we have the value for x, we can plug it into the second equation to easily find y.
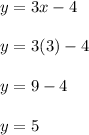
Finally, we can plug x and y into the first equation in order to check to make sure it works for the other equation
