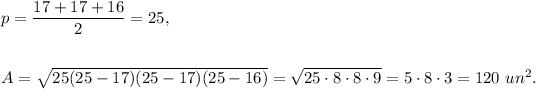Question

Given: ΔPSQ, PS = SQ PΔPSQ = 50 SQ – PQ = 1 Find: Area of ΔPSQ

Answer 1

120 square units

Explanation:

In triangle PSQ, PS=SQ. Let PS=SQ=x units.

Since SQ-PQ=1, PQ=SQ-1=x-1 units.

The perimeter of the triangle PSQ is 50 units, so

PS+SQ+PQ=50 units.

Substitute PS=SQ=x un. and PQ=x-1 un.

x+x+x-1=50

3x=51

x=17

Hence

PS=SQ=17 units,

PQ=16 units.

Use Heron's formula to find the area:

`A=√(p(p-a)(p-b)(p-c)),`

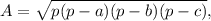
where p is semi-perimeter and a,b,c are lengths of sides.

`p=(17+17+16)/(2)=25,\\ \\\\A=√(25(25-17)(25-17)(25-16))=√(25\cdot 8\cdot 8\cdot 9)=5\cdot 8\cdot 3=120\ un^2.`