Answer with step-by-step explanation:
We are given the following two functions:
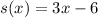
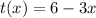
We are to find the simplified formula and domain for
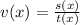 and
and
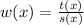 .
.
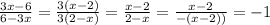
So the domain for this function is (-∞, +∞) for v(x) = -1.
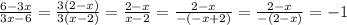
The domain of this function is (-∞, +∞) for w(x)= -1