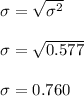Answer: Second Option
Explanation:
We have the average January surface water temperatures (°C) of Lake Michigan from 2000 to 2009.
5.07, 3.57, 5.32, 3.19, 3.49, 4.25, 4.76, 5.19, 3.94, 4.34
We know that the average
 of the data is:
of the data is:
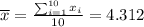
We also know that the variance
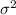 is equal to 0.577.
is equal to 0.577.
So by definition the standard deviation
 is:
is: