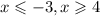Question

What is the solution of the inequality (x-4)(x+3) more then or equal to zero? Graph Solution.

Answer 1

`x \leqslant - 3,x \geqslant 4`

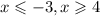
EXPLANATION

The given inequality is

`(x - 4)(x + 3) \geqslant 0`

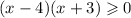
To solve this inequality, we need to solve the corresponding equation:

`(x - 4)(x + 3) = 0`

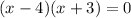
This implies that

`x = - 3 \: or \: x = 4`

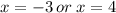
We now plot the solutions of the equation and use it to solve the corresponding inequality as shown in the attachment.

These values divide the number line into 3 regions.

The region(s) that satisfies the inequality is the solution set

From the graph the solution is

`x \leqslant - 3,x \geqslant 4`