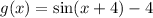Question

What sine function represents an amplitude of 1, a period of 2pi, a horizontal shift of our, and a vertical shift of -4

Answer 1

`g(x) = \sin(x +4) - 4`

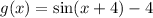
Step-by-step explanation

The basic sine function is

`f(x) = \sin(x)`

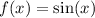
The transformed sine function has equation

`g(x) = a \sin(bx +c ) + d`

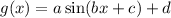
where a=1 is the amplitude

2π/b=2π is the period

c/b= 4 is the horizontal shift 4 units left.

d=-4 is a downward vertical shift of 4 units.

The required sine function is:

`g(x) = \sin(x +4) - 4`