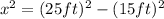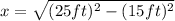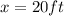Question

A 25-foot ladder leans against a wall. The base of the ladder is 15 feet from the bottom of the wall. How far up the wall does the top of the ladder reach?

Answer 1

Answer: 20 feet.

Explanation:

Observe the right triangle attached.

You need to find the value of "x".

Then, you can use the Pythagorean Theorem:

`a^2=b^2+c^2`

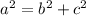
Where "a" is the hypotenuse of the triangle, and "b" and "c" are the legs.

In this case, you can identify that:

`a=25ft\\b=15ft\\c=x`

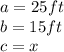
Substitute these values into
`a^2=b^2+c^2`:

`(25ft)^2=(15ft)^2+x^2`

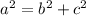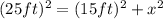
Now, you need to solve for x to find how far up the wall the top of the ladder reaches. Then you get:

`x^2=(25ft)^2-(15ft)^2`

`x=√((25ft)^2-(15ft)^2)`

`x=20ft`

Answer 2

20ft

EXPLANATION

The ladder, the wall and the ground formed a right triangle.

Let how far up the wall does the top of the ladder reached be x units.

The 25ft ladder is the hypotenuse.

The shorter legs are, 15ft and x ft

Then from Pythagoras Theorem,

`{x}^(2) + {15}^(2) = {25}^(2)`

`{x}^(2) + 225 = 625`

`{x}^(2)= 625 - 225`

`{x}^(2)= 400`

`x = √(400)`

`x = 20ft`

Therefore the ladder is 20 ft up the wall.