Question

What is the inverse of the following function?
y= 2^x + 3

Answer 1

Option B:
`y=log_2(x-3)`

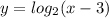
Explanation:

To obtain the inverse of the function
`y= 2^x + 3`, you need to solve the equation for the variable "x":

`y= 2^x + 3\\\\y-3=2^x\\\\log_2(y-3)=log_2(2^x)\\\\x=log_2(y-3)`

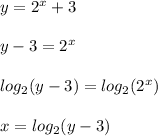
Now you need to exchange the variable "x" with the variable "y". Then:

`y=log_2(x-3)`

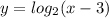
Finally, you get that the inverse of the function
`y= 2^x + 3` is:

`y=log_2(x-3)`

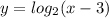
This matches with the option B.