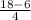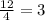Answer:
3
Explanation:
The given function is
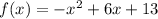
The average rate of change is simply the slope of the secant line connecting any two point on the graph of the function.
The average rate of change of this function over the interval;
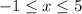 is given by:
is given by:
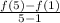
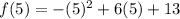
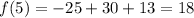
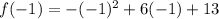

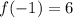
The average rate of change now becomes;