We have
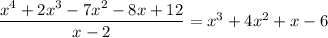
The rational root theorem suggests that other possible roots may be -6, 6, -3, 3, -2, 2, -1, and 1. It turns out that
 is a root, since
is a root, since
 , so
, so
 is also a factor and we have
is also a factor and we have
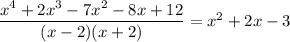
Finally, we can factorize the remaining quotient easily:

so the other factors are
 ,
,
 , and
, and
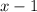 .
.