Question

Solve the following quadratic equation for x by completing the square and solve x^2-8x=-65​

Answer 1

x = 4+7i and x=4-7i

Explanation:

I just did the test

Answer 2

x= 4-7i x= 4+ 7i

Explanation:

step 1 : make equation = 0

`x^(2)` - 8x + 65 = 0

step 2 : solve for x [ using the quadratic equation]

ie : x = -b ±
`\sqrt{b^(2)-4ac}` / 2a

so it will look like this

x= -(-8) ±
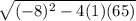`\sqrt{(-8)^(2) - 4(1)(65)}` /2(1)

when you simplify you wont be able to root the -196 so you will have to separate the roots

x = 8 ±(
`√(-1)` )(
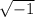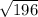`√(196)`) / 2

now there is a rule for negative roots whereby
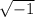`√(-1)` is equivalent to i so now you will change
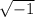`√(-1)` into i

Simplify
`√(196)`

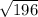
which will give you 14

now place all the new values into the formula

8 ± 14i /2

you can then further simplify to

4 ± 7i

step 3 : separate

this will give you the final answer of

x= 4 + 7i x= 4- 7i