Answer:
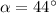
Explanation:
By definition, the tangent of an angle is the quotient between the side opposite the angle and the side adjacent to the angle
In other words:
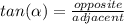
In this triangle, the length of the side adjacent to the desired angle is 50, and the length of the opposite side is 48
So:
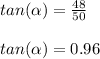
Finally
