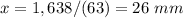Answer:
The height of the triangular prism is
Explanation:
see the attached figure to better understand the problem
we know that
The volume of the triangular prism is equal to
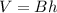
where
B is the area of the triangular base
h is the height of the prism
Find the area of the base B
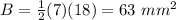
we have
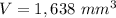
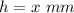
substitute and solve for x