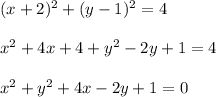Question

What is the general form of the equation of a circle with its center at (-2, 1) and passing through (-4, 1)?

Answer 1

`x^(2)+y^(2) +4x-2y+1=0`

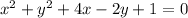
Explanation:

we know that

The general form of the equation of a circle is

`x^(2) +y^(2)+ Dx + Ey + F=0`

where D, E, F are constants

step 1

Find the radius of the circle

Remember that the distance from the center to any point on the circle is equal to the radius

so

the formula to calculate the distance between two points is equal to

`d=\sqrt{(y2-y1)^(2)+(x2-x1)^(2)}`

`(-2,1)\\(-4,1)`

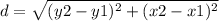
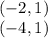
substitute the values

`r=\sqrt{(1-1)^(2)+(-4+2)^(2)}`

`r=\sqrt{(0)^(2)+(-2)^(2)}`

`r=2\ units`

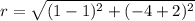
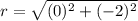
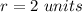
step 2

Find the equation of the circle in standard form

`(x-h)^(2) +(y-k)^(2)=r^(2)`

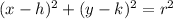
In this problem we have

center ( -2,1)

radius r=2 units

substitute

`(x+2)^(2) +(y-1)^(2)=2^(2)`

`(x+2)^(2) +(y-1)^(2)=4`

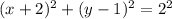
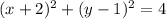
Step 3

Convert to general form

`(x+2)^(2) +(y-1)^(2)=4\\ \\x^(2)+4x+4+y^(2)-2y+1=4\\ \\x^(2)+y^(2) +4x-2y+1=0`